Question

Given the rational function below and it’s graph, explain why there is a “hole” in the graph at x=2 hints: are there any inputs that are undefined? Can you factor the numerator? Does it simplify? Does it tell you why this graphs as a line?

Answer 1

Explanation:

Given function is,
`f(x)=(x^2-3x+2)/(x-2)`

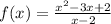
Factors of the numerator = x² - 3x + 2

= x² - 2x - x + 2

= x(x - 2) - 1(x - 2)

= (x - 2)(x - 1)

Therefore, given function will be,

f(x) =
`((x-2)(x-1))/((x-2))`

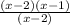
For (x - 2) = 0

For x = 2, denominator will become zero.

Thus for input value x = 2, function will not be defined.

If x ≠ 2, f(x) = x - 1

It's a linear function represented by f(x) = x - 1

Having slope = 1 and y-intercept = -1

And (2, 1) is a hole as shown in the graph.