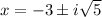Question

What is the roots of f(x)=-x^2-6x-14

Answer 1

`x=-3\pm i √(5)`

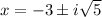
General Formulas and Concepts:

Pre-Algebra

• Order of Operations: BPEMDAS
• Equality Properties

Algebra I

• Standard Form: ax² + bx + c = 0
• Quadratic Formula:
  `x=(-b\pm√(b^2-4ac) )/(2a)`

Algebra II

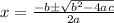
• Imaginary roots: √-1 = i

Explanation:

Step 1: Define function

f(x) = -x² - 6x - 14

Step 2: Set up

1. Set equation equal to 0: -x² - 6x - 14 = 0
2. Factor out -1: -(x² + 6x + 14) = 0
3. Divide both sides by -1: x² + 6x + 14 = 0

Step 3: Define variables

a = 1

b = 6

c = 14

Step 4: Find roots

1. Substitute:
   `x=(-6\pm√(6^2-4(1)(14)) )/(2(1))`
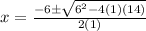
2. Exponents:
   `x=(-6\pm√(36-4(1)(14)) )/(2(1))`
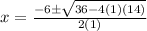
3. Multiply:
   `x=(-6\pm√(36-56) )/(2)`
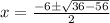
4. Subtract:
   `x=(-6\pm√(-20) )/(2)`
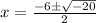
5. Factor:
   `x=(-6\pm√(-1) √(20) )/(2)`
6. Simplify:
   `x=(-6\pm2i √(5) )/(2)`
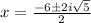
7. Factor:
   `x=(2(-3\pm i √(5) ))/(2)`
8. Divide:
   `x=-3\pm i √(5)`