Question

Which function is the inverse of f(x)=x²-16 if the domain is f(x)>0?​

Answer 1

`f^(-1)(x)=√(x+16)`

Explanation:

`f(x)=x^2-16`

The inverse of the function
`f` is
`f^(-1)`

The Range of the inverse function
`f` will be the Domain of the function
`f`

`Ran(f^(-1)) = [0, \infty)`

The inverse of

`f(x)=x^2-16`

is

`f^(-1)(x)=√(x+16)`

Note that if the domain of the function
`f` where all the Real numbers, the inverse would be

`f^(-1)(x)=\pm√(x+16)`

Answer 2

Inverse of
`f(x)=x^2-16` is
`f^(-1)(x)=√(x+16)`

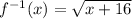
Option A is correct option.

Explanation:

We need to find inverse of
`f(x)=x^2-16`

For finding inverse replace f(x) with y

`y=x^2-16`

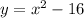
Now, solve for x

Adding 16 on both sides

`y+16=x^2-16+16\\y+16=x^2\\=> x^2=y+16`

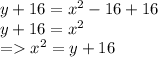
Taking square root on both sides:

`x^2=y+16\\√(x^2) =√(y+16) \\x=√(y+16)`

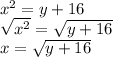
Now replace x with f^{-1}(x) and y with x

`f^(-1)(x)=√(x+16)`

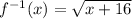
So, inverse of
`f(x)=x^2-16` is
`f^(-1)(x)=√(x+16)`

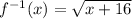
Option A is correct option.