Answer:
Inverse of f(x)=-x^3-9 is
![f^(-1)(x)=\sqrt[3]{-x-9}](https://img.qammunity.org/2021/formulas/mathematics/high-school/cr9fb7f4k85j5as1o3ou02mi50k741lebz.png)
Option B is correct option.
Explanation:
We need to find inverse of

For finding the inverse replace f(x) with y
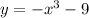
Now, solve for x
Adding 9 on both sides
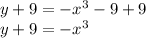
Multiply both sides by -1
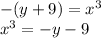
Taking cube root on both sides:
![x^3=-y-9\\\sqrt[3]{x^3} =\sqrt[3]{-y-9} \\x=\sqrt[3]{-y-9}](https://img.qammunity.org/2021/formulas/mathematics/high-school/potpw2t3bncelvzlp1q5s61gfd60we05eo.png)
Now replace x with f^{-1}(x) and y with x
![f^(-1)(x)=\sqrt[3]{-x-9}](https://img.qammunity.org/2021/formulas/mathematics/high-school/cr9fb7f4k85j5as1o3ou02mi50k741lebz.png)
So, inverse of f(x)=-x^3-9 is
![f^(-1)(x)=\sqrt[3]{-x-9}](https://img.qammunity.org/2021/formulas/mathematics/high-school/cr9fb7f4k85j5as1o3ou02mi50k741lebz.png)
Option B is correct option.