Answer:
Choice B
Explanation:
The best way to compare two lines is to solve for y.
The first equation:
For
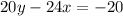 the first thing we should do is add the 24x on both sides which will cancel out itself on the left side
the first thing we should do is add the 24x on both sides which will cancel out itself on the left side
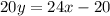 . Now all we have to do is divide the 20 on both sides to leave us with
. Now all we have to do is divide the 20 on both sides to leave us with
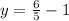 .
.
The second equation:
For
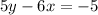 the first thing we should do is add the 6x on both side which will cancel itself on the left side
the first thing we should do is add the 6x on both side which will cancel itself on the left side
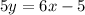 . Now all we have to do is divide the 5 on both sides to leave us with
. Now all we have to do is divide the 5 on both sides to leave us with
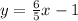 .
.
Now for the comparison:
They are the same exact line. If you were to graph them they would overlap each other.