Answer:
Given equation:
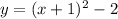
The function is a quadratic function in vertex form:

Translations
For
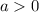
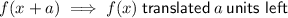

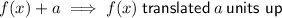

Parent function:

Translations
Translated 1 unit left:
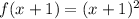
Then translated 2 units down:
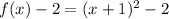
Therefore, translate the parent function by 1 unit left and 2 units down to produce the given equation.