Question

The current through a 0.1 Henrys (H) inductor is i(t) = 10 t e^-5tA. Find the voltage across the inductor.

a. v(t) = 10 te^-5t V
b. v(t) = 0.1 (10te^-5t) V
c. v(t) = 5te^-5t V
d. v(t) = (1 - 5t) e^-5t V

Answer 1

Answer: d.
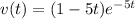`v(t)=(1-5t)e^(-5t)`V

Step-by-step explanation: Inductance is a property of an inductor: when there is a change in current passing through a conductor, it creates a voltage in the conductor itself and in the other conductors. Inductance unit is Ω.s or henry (H)

So, the relation between Voltage and Current in an inductor is given by

`v=L(di)/(dt)`

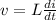
in which

L is inductance in H

i is current in A

Current is
`i(t)=10te^(-5t)`, so, derivative will be:

`(di)/(dt)=10e^(-5t)+10t(-5)e^(-5t)`

`(di)/(dt)=10e^(-5t)-50te^(-5t)`

`(di)/(dt)=10e^(-5t)(1-5t)`

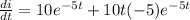
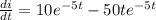
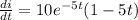
Then, voltage is

`v=0.1.10.e^(-5t)(1-5t)`

`v=(1-5t)e^(-5t)`

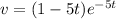
Voltage across the 0.1H inductor is
`(1-5t)e^(-5t)` V