Answer:
Chase and his brother will both have a score of 31 points by making 4 successful shots.
Explanation:
Given that initially Chase has 19 points and his brother has 27 points.
In one successful flip, Chase get 3 points and in one successful flip, his little brother get 3 points
Assuming that both have the same score after n number successful flip of the coin.
So, points earned by Chase in n successful flip
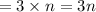
and points earned by his little brother in n successful flip
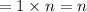 .
.
Total points of Chase
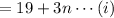
Total points of his little brother
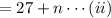
As the brothers have tied scores in the game, that means both have the same score, so from equations (i) and (ii), we have
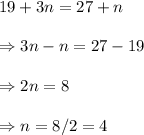
So, after n successful throw, both have the same score.
Hence, each brother made 4 additional successful shots.
Now, put n=4 inequation (i) or (ii) to get the total points, i.e
Total points of each brother

Hence, each brother has 31 points.