Question

Help I dont know how to do this without the y intercept ​

Answer 1

Answer: y = (2/3)x + 4/3

Slope = 2/3

Y intercept = 4/3

======================================================

Step-by-step explanation:

First we need the slope

The two blue points on this line are (1,2) and (4,4)

Let's say (x1,y1) = (1,2) and (x2,y2) = (4,4)

Use the slope formula

m = (y2-y1)/(x2-x1)

m = (4-2)/(4-1)

m = 2/3

Note how we go up 2 units and then to the right 3 units when going from the point (1,2) to the point (4,4)

In other words, slope = rise/run = 2/3 breaks down to rise = 2 and run = 3

rise = 2 = go up 2

run = 3 = go to the right 3

--------------------------------

Now we'll use point slope form

y - y1 = m(x - x1)

to get the following

y - y1 = m(x - x1)

y - y1 = (2/3)(x - x1) .... plug in m = 2/3

y - 2 = (2/3)(x - 1) ...... plug in (x1,y1) = (1,2)

Now we solve for y

y - 2 = (2/3)(x - 1)

y - 2 = (2/3)(x) + (2/3)(-1)

y - 2 = (2/3)x - 2/3

y = (2/3)x - 2/3 + 2 ... adding 2 to both sides

y = (2/3)x - 2/3 + 6/3 .... rewrite 2 as 6/3

y = (2/3)x + (-2+6)/3

y = (2/3)x + 4/3

This is the same as writing
`y = (2)/(3)x + (4)/(3)`

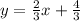
This equation is in the form y = mx+b with

m = 2/3 = slope

b = 4/3 = y intercept

Answer 2

`y=(2)/(3) x+(4)/(3)`

Step-by-step explanation:

We have two points (1, 2) and (4, 4).

Let's start with y = mx + b

Plug in each point.

2 = m + b

4 = 4m + b

Subtract the first from the second.

2 = 3m

m = 2/3

Plug m back in the fist.

2 = 2/3 + b

b = 4/3