Answer:

Explanation:
The standard form of a circle is given by the equation:

Where (h, k) is the center and r is the radius.
We know that the center is (2, -5). So, we can substitute 2 for h and -5 for k. This yields:
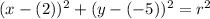
Simplify:
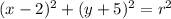
Now, we need to determine r. We know that a point on the circle is (-4, -5). Thus, we can determine r by substituting -4 for x and -5 for y. This yields:
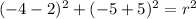
Evaluate:
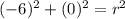
Evaluate:
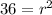
Hence, the radius squared is 36.
We do not actually need to solve for r, as we will square it anyways.
We will substitute 36 for r squared. Hence, our equation is:

The radius will thus be 6 units.