Problem 1
Any pair of equilateral triangles are always similar triangles. We can use the SSS (side side side) similarity theorem to prove this. One triangle is an enlarged copy of the other, or a shrunken reduced copy.
The rectangle is NOT similar to the square. They are different shapes. Similar figures are figures that are the same shape, but different sizes.
==========================================================
Problem 2
horizontal/vertical = horizontal/vertical
24/x = 6/5
24*5 = 6x
120 = 6x
x = 120/6
x = 20
Answer: The flagpole is 20 ft high (about 2 stories tall)
==========================================================
Problem 3
The formula to use is
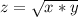
z = geometric mean of x and y
We multiply the numbers and take the square root. This only works for two values at a time. The geometric mean of three numbers will involve the cube root. Four numbers involves the 4th root, and so on.
- Geometric mean of 6 and 10 is sqrt(60) = 7.74597 approximately
- Geometric mean of 2 and 32 is 8 exactly
- Geometric mean of 2 and 8 is 4 exactly
- Geometric mean of 7 and 9 is sqrt(63) = 7.93725 approximately
==========================================================
Problem 4
For each figure, the y is the altitude and can be determined using the geometric mean formula.
- Figure on the left: y = sqrt(2*6) = sqrt(12) = 3.4641
- Figure on the right: y = sqrt(6*14) = sqrt(84) = 9.1652
The decimal values are approximate.
==========================================================
Problem 5
For 45-45-90 triangles, we divide the hypotenuse over sqrt(2) to find the leg length.
For the first triangle in the first row, we have y = 18/sqrt(2) = 9*sqrt(2) after rationalizing the denominator. Multiply top and bottom by sqrt(2).
Take this process in reverse to determine the hypotenuse if you know the leg length. This explains why the hypotenuse is 3.2*sqrt(2) for the second triangle in the first row.
-------------------
For 30-60-90 triangles, we go from the short leg x to the hypotenuse 2x.
Double the short leg to get the hypotenuse.
2x = 36
x = 36/2
x = 18
This is for the first triangle in the bottom row.
For the other triangle in this bottom row, we have a long leg of 4*sqrt(3). This must mean the short leg is exactly 4 units long. Therefore, the hypotenuse is 2*4 = 8 units long.
-------------------
Summary:
- Top row, first column: y = 9*sqrt(2)
- Top row, second column: y = 3.2*sqrt(2)
- Bottom row, first column: x = 18
- Bottom row, second column: y = 8