Answer:
Average rate of change = 0.4
Explanation:
Average rate of change of a function in the interval [a, b] is defined by the formula,
Rate of change =

From the table attached,
Rate of change of the function in the interval 20 ≤ x ≤ 40 will be
=
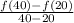
=
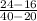
=
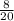
=

= 0.4
Therefore, average rate change of the function in the given interval 20 ≤ x ≤ 40 is = 0.4