Answer:
A) 84 cubes
B) Volume = 4 x 3 x 7 = 84 small cubes
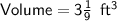
Explanation:
Part A
As the lengths of each side of the small cube are ¹/₃ ft each, to find the number cubes in the rectangular prism, first find how many thirds are in each dimension of the prism:
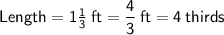

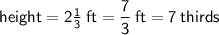
Now simply multiply the number of thirds:
Number of cubes in prism = 4 x 3 x 7 = 84
Part B
As we have already found the dimensions of the prism in terms of the number of cubes, the volume of the prism in terms of the small cube is:
⇒ Volume = 4 x 3 x 7 = 84 small cubes
To find the volume of the prism in ft³, calculate the actual volume of the small cube:
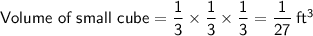
Now multiply the volume in terms of number of cubes by the actual volume of a cube:
