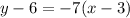Question

Complete the point-slope equation of the line through (3,6)(3,6)left parenthesis, 3, comma, 6, right parenthesis and (5,-8)(5,−8)left parenthesis, 5, comma, minus, 8, right parenthesis.

Answer 1

The required equation of line in point-slope form is:

`y-6 = -7(x-3)`

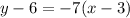
Explanation:

Given points are:

(x1,y1) = (3,6)

(x2,y2) = (5,-8)

The point-slope form of an equation is given by:

`y-y_1 = m(x-x_1)`

Here m is slope of the line and (x1,y1) is a point on line

The slope is calculated using the formula:

`m = (y_2-y_1)/(x_2-x_1)`

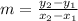
Putting the values we get

`m = (-8-6)/(5-3)\\m=(-14)/(2)\\m= -7`

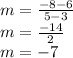
Putting in the equation

`y-y_1 = -7(x-x_1)`

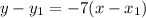
Now we have to put a point in the equation, putting (3,6) in the equation

`y-6 = -7(x-3)`

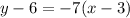
Hence,

The required equation of line in point-slope form is:

`y-6 = -7(x-3)`