Question

a shipping crate is a perfect cube with volume of 2,197 cubic feet. the ceiling of the warehouse is 14 feet high. will the crate fit in the warehouse explain why

Answer 1

As the height of warehouse is greater than the height of crate, the crate will fit in the warehouse.

Explanation:

Given that

Volume of cube = V = 2197 cubic feet

Side of cube = a =?

Height of ware house = h = 14 feet

In order for the crate to fit in the warehouse the height of crate has to be less than the height of warehouse i.e. a<h

In order to check this we have to find the side/height of crate

So,

`V = a^3\\2197 = a^3`

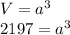
Taking cube root on both sides

`\sqrt[3]{a^3} = \sqrt[3]{2197}\\\sqrt[3]{a^3} = \sqrt[3]{13^3}\\ a = 13\ feet`

So the height of cube is 13 feet.

Comparing the height of c and height of ware house we can conclude that

Height of Warehouse > Height of crate

h>a

14>13

As the height of warehouse is greater than the height of crate, the crate will fit in the warehouse.