(3) Differentiating both sides of

with respect to x gives

Solve for dy/dx :
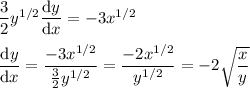
Then the slope of the tangent line to the curve at (1, 9) is

The equation of the tangent line would then be
y - 9 = -2/3 (x - 1) ==> y = -2/3 x + 29/3
(4) The slope of the tangent line to
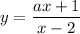
at a point (x, y) on the curve is
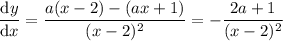
When x = -1, we have a slope of 2/3, so
-(2a + 1)/(-1 - 2)² = 2/3
Solve for a :
-(2a + 1)/9 = 2/3
2a + 1 = -18/3 = -6
2a = -7
a = -7/2