Answer:
The solution of this differential system is
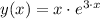 .
.
Explanation:
This second-order differential equation is homogeneous and linear of the form:
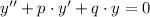 (1)
(1)
Where:
 - First-order constant coefficient, dimensionless.
- First-order constant coefficient, dimensionless.
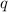 - Zero-order constant coefficient, dimensionless.
- Zero-order constant coefficient, dimensionless.
Whose characteristic polynomial is:
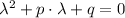 (2)
(2)
Where
 contains the roots associated with the solution of the differential equation.
contains the roots associated with the solution of the differential equation.
If we know that
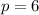 and
and
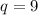 , the roots of the characteristic equation are, respectively:
, the roots of the characteristic equation are, respectively:

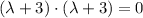
Which means that
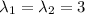 and the solution of the differential equation is of the form:
and the solution of the differential equation is of the form:
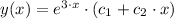 (3)
(3)
Where
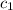 and
and
 are integration constants.
are integration constants.
The first derivative of the equation above is:
 (4)
(4)
Now, if we get that
 and
and
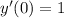 , then the system of equations to the solved is:
, then the system of equations to the solved is:
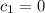 (3b)
(3b)
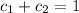 (4b)
(4b)
The solution of this system is:
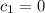 ,
,
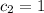 . Therefore, the solution of this differential system is
. Therefore, the solution of this differential system is
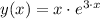 .
.