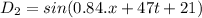Answer:
The equation for such type of wave is
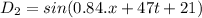
Step-by-step explanation:
From the question we are told that
The displacement is

Here the wave number is k= 0.84
The angular frequency is

The phase shift is
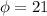
Generally the equation that represents a wave which, when traveling in the opposite direction, will produce a standing wave when added to this one is mathematically represented as