Question

Which of the following could represent the unit side lengths of a right triangle

A 5,8,10

B 3,5,7

C 6,11,13

D 12,16,20

Answer 1

Choice D

Explanation:

If you check each one plugging in your info into this you can get
`a^(2) +b^(2) =c^(2)` that 12² + 16² will equal 400 which is the same as 20².

Answer 2

Answer: D 12,16,20

Explanation:

The Pythagora's Theorem

On a right triangle with side lengths of a,b, and c, being c the hypotenuse, of the longest side, it follows that:

`a^2+b^2=c^2`

The set {a,b,c} is often called a Pythagorean Triple.

Testing every option:

A

`5^2+8^2=10^2`

`25+64 = 100`

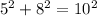
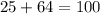
89 = 100

Since equality is false, 5,8,10 are not the side lengths of a right triangle.

B

`3^2+5^2=7^2`

`9+25 = 49`

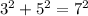
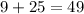
34 = 49

Since equality is false, 3,5,7 are not the side lengths of a right triangle.

C

`6^2+11^2=13^2`

`36+121 = 169`

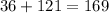
157 = 169

Since equality is false, 6,11,13 are not the side lengths of a right triangle.

D

`12^2+16^2=20^2`

`144+256 = 400`

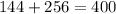
400 = 400

Since equality is true, 12,16,20 could be the side lengths of a right triangle.

Answer: D 12,16,20