Question

A 10 ft ladder is being pulled away from a wall at a rate of 3 ft/sec. What is the rate of change in the area beneath the ladder when the ladder is 6 ft from the wall

Answer 1

The rate of change in the area beneath the ladder is 5.25 ft²/s

Explanation:

Area of triangle is given by;

`A = (1)/(2)bh\\\\2A = bh\\\\take \ derivative \ of \ both \ sides \ with \ respect \ to \`

where;

b is the base of the triangle, given as 6 ft

h is the height of the triangle, determined by applying Pythagoras theorem.

h² = 10² - 6²

h² = 100 - 36

h² = 64

h = √64

h = 8 ft

Determine the rate of change of the height;

`h^2 + b^2 = 10^2\\\\h^2 + b^2 =100\\\\2h(dh)/(dt) + 2b(db)/(dt) =0\\\\h(dh)/(dt) + b(db)/(dt) =0\\\\h (dh)/(dt) = -b(db)/(dt) \\\\(dh)/(dt) = ((-b)/(h) )(db)/(dt)\\\\(dh)/(dt) =((-6)/(8))(3)\\\\(dh)/(dt) = -(9)/(4) \ ft/s`

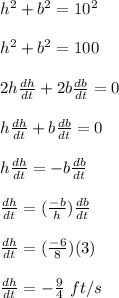
Finally, determine the rate of change of area beneath the ladder;

`(dA)/(dt) = ((h)/(2) )(db)/(dt) + ((b)/(2)) (dh)/(dt)\\\\(dA)/(dt) = ((8)/(2) )(3) + ((6)/(2)) ((-9)/(4))\\\\(dA)/(dt) = 12 - (27)/(4) \\\\(dA)/(dt) = (48-27)/(4)\\\\(dA)/(dt) = (21)/(4) \ ft^2/s\\\\(dA)/(dt) = 5.25 \ ft^2/s`

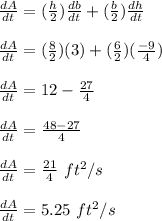
Therefore, the rate of change in the area beneath the ladder is 5.25 ft²/s