Answer:
Length of the rectangle is 8 in and the breadth is 8 in.
a.
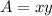
b.
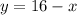
Explanation:
Length = x
Breadth = y
Area is given by
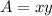
Perimeter of rectangle is given by
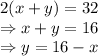
The condition that x and must satisfy is
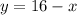
So, area is
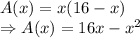
Differentiating with respect to x we get
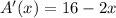
Equating with zero
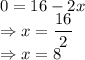
Double derivative of A(x)
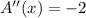
So
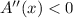 which means
which means
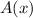 is maximum at
is maximum at
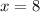

So length of the rectangle is 8 in and the breadth is 8 in.