Answer:
11.55 years
Explanation:
Formula to calculate the final value when the money is compounded continuously is,
Final value =
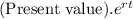
Here 'r' = rate of interest
t = duration for the investment
Let the money invested = $x
We have to find the duration in which the present value gets doubled.
Final value = $2x
Rate of interest = 6% per year ≈ 0.06
By substituting these values in the formula,

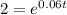
ln(2) =
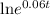
0.693147 = 0.06t
t = 11.55 years
Therefore, invested amount will be doubled in 11.55 years.