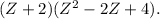Question

Factor using the identity:
a3 + b3 = (a + b)(a? - ab + b2)
Z3+8

Answer 1

The given expression Z^3 + 8 can be factored as (Z + 2)(Z^2 - 2Z + 4).

Step-by-step explanation:

A mathematical expression is a combination of numbers, variables, and operations such as addition, subtraction, multiplication, and division. It represents a concise way to describe a mathematical relationship or calculation, providing a clear and structured representation of a mathematical idea or problem.

The given expression
`Z^3 + 8.`

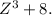
This can be factored using the identity
`a^3 + b^3 = (a + b)(a^2 - ab + b^2).`

Substituting a = Z and b = 2 in the identity, we have:

`Z^3 + 8 = (Z + 2)(Z^2 - Z*2 + 2^2).`

So, the factored form of the given expression is
`(Z + 2)(Z^2 - 2Z + 4).`