Answer:
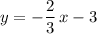 .
.
Explanation:
Rewrite the equation
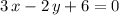 in the slope-intercept form
in the slope-intercept form
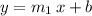 to find the slope of this given line:
to find the slope of this given line:
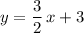 .
.
Thus, the slope of this given line is
 .
.
Let
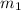 and
and
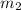 denote the slope of the given line and the slope of line
denote the slope of the given line and the slope of line
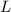 , respectively. Two lines in a plane are perpendicular to one another if and only if the product of their slopes is
, respectively. Two lines in a plane are perpendicular to one another if and only if the product of their slopes is
 . Therefore, for these two lines to be perpendicular to one another,
. Therefore, for these two lines to be perpendicular to one another,
 .
.
Since
 according to the equation of the given line, the slope of line
according to the equation of the given line, the slope of line
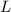 would be:
would be:
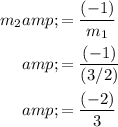 .
.
If a line in a plane has slope
 and goes through the point
and goes through the point
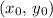 , the slope-point equation of that line would be
, the slope-point equation of that line would be
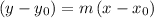 .
.
Since the line
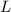 goes through
goes through
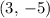 , the equation of this line in slope-point form would be:
, the equation of this line in slope-point form would be:
 .
.
Rearrange to find the equation of line
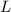 in slope-intercept form:
in slope-intercept form:
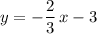 .
.