a. If A is the coefficient matrix, solve det(A - λI) = 0 for the eigenvalues λ :
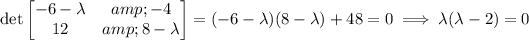
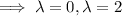
Let v = [v₁, v₂]ᵀ be the eigenvector corresponding to λ. Solve Av = λv for v :

If we pick v₂ = -3, then v₁ = 2, so [2, -3]ᵀ is the eigenvector for λ = 0.

Let v₁ = 1, so v₂ = -2.
b. λ = 0 and v = [2, -3]ᵀ contributes a constant solution,
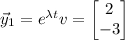
while λ = 2 and v = [1, -2]ᵀ contribute a solution of the form
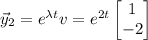
c. Yes; compute the Wronskian of the two fundamental solutions:

The Wronskian is non-zero, so the solutions are independent.