Answer:
The first step is to divide all the terms by the coefficient of
 which is 2.
which is 2.
The solutions to the quadratic equation
 are:
are:

Explanation:
Considering the equation

The first step is to divide all the terms by the coefficient of
 which is 2.
which is 2.
so
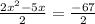
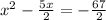
Lets now solve the equation by completeing the remaining steps
Write equation in the form:
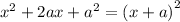
Solving for
 ,
,
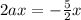
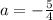
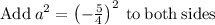
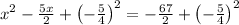

Completing the square
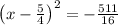
Since, you had required to know the first step in completing the square for the equation above, I hope you have got the point, but let me quickly solve the remaining solution.
For
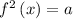 the solution are
the solution are
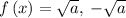
Solving
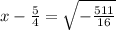

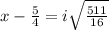 ∵ Applying imaginary number rule
∵ Applying imaginary number rule


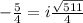
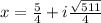
Similarly, solving
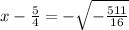
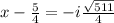 ∵ Applying imaginary number rule
∵ Applying imaginary number rule

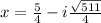
Therefore, the solutions to the quadratic equation are:
