Question

David created two functions. Function A can be represented by the equation y= 3/4x + 2

Function B can be represented by the graph below.

Which of the following statements correctly compares the two functions.

Function A has the greater rate of change and the greater y-intercept.

Function B has the greater rate of change and the greater y-intercept.

Function A has the greater rate of change and Function B has the greater y-intercept.

Function B has the greater rate of change, and Function A has the greater y-intercept.

Answer 1

Function B has the greater rate of change, and Function A has the greater y-intercept

Explanation:

Equation representing Function A: y= 3/4x + 2

Where,

slope/rate of change (m) = ¾

y-intercept (b) = 2

Using the graph given for Function B, let's find an equation for Function B by determining the slope of the line/rate of change (m) and y-intercept (b).

Using points (1, 0) and (2, 2), which are two points on the line, find slope (m), which is also represents the rate of change.

`m = (y_2 - y_1)/(x_2 - x_1) = (2 - 0)/(2 - 1) = (2)/(1) = 2`

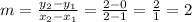
Rate of change for function B (m) = 2

y-intercept (b) is the point at which the y-axis is intercepted by the line of the graph = -2

Substitute, m = 2, and b = -2 into y = mx + b.

The equation for Function B would be:

y = 2x +(-2)

y = 2x - 2.

slope/rate of change (m) = 2

y-intercept (b) = -2

Comparing the Function A and Function B,

Function A rate of change is ¾, while that of B is 2. Therefore, Function B has the greater rate of change.

Function A has y-intercept of 2, while Function B has a y-intercept of -2. Therefore, Function A has the greater y-intercept.