Question

The area of a rectangular shop in the mall is 160 square meters. The perimeter is 52 meters. What are the dimensions of the shop?

Answer 1

width = 10 m

length = 16 m

Explanation:

Formula

`\textsf{Area of a rectangle} = wl`

`\textsf{Perimeter of a rectangle}=2(w+l)`

(where
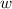`w` is width and
`l` is length)

Given:

• Area = 160 m²
• Perimeter = 52 m

Substituting the given values into the formulae to create two equations:

`\textsf{Equation 1}: \quad wl=160`

`\textsf{Equation 2}: \quad 2(w+l)=52`

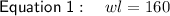
Rearranging Equation 1 to make w the subject:

`\implies w=(160)/(l)`

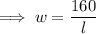
Substituting expression for w into Equation 2 and solving for
`l`:

`\implies 2\left((160)/(l)+l\right)=52`

`\implies (160)/(l)+l=26`

`\implies 160+l^2=26l`

`\implies l^2-26l+160=0`

`\implies l^2-10l-16l+160=0`

`\implies l(l-10)-16(l-10)=0`

`\implies (l-10)(l-16)=0`

`\implies l=10, 16`

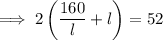
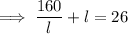
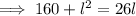
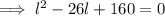
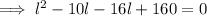
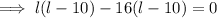
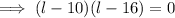
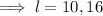
According to Equation 1:

• If length = 10 m ⇒ width = 16 m
• If length = 16 m ⇒ width = 10 m

As width < length, the dimensions of the shop are:

• width = 10 m
• length = 16 m