Answer:
The modulus of resilience is
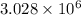 joules per cubic meter.
joules per cubic meter.
Step-by-step explanation:
At first we assume that allow behaves elastically, then the formula for the modulus of resilience (
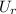 ), measured in joules per cubic meter, is:
), measured in joules per cubic meter, is:
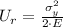 (Eq. 1)
(Eq. 1)
Where:
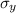 - Yield strength, measured in pascals.
- Yield strength, measured in pascals.
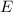 - Modulus of elasticity, measured in pascals.
- Modulus of elasticity, measured in pascals.
If we know that
 and
and
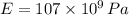 , then the modulus of resilience is:
, then the modulus of resilience is:
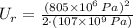

The modulus of resilience is
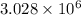 joules per cubic meter.
joules per cubic meter.