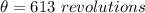Complete Question
The angular speed of an automobile engine is increased at a constant rate from 1120 rev/min to 2560 rev/min in 13.8 s.
(a) What is its angular acceleration in revolutions per minute-squared
(b) How many revolutions does the engine make during this 20 s interval?
rev
Answer:
a
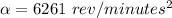
b
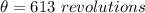
Step-by-step explanation:
From the question we are told that
The initial angular speed is

The angular speed after
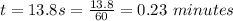 is
is
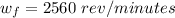
The time for revolution considered is
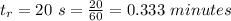
Generally the angular acceleration is mathematically represented as

=>
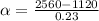
=>
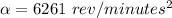
Generally the number of revolution made is
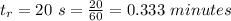 is mathematically represented as
is mathematically represented as
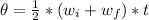
=>
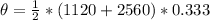
=>