Answer:
Given inequalities:
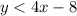
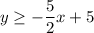
Part A
The graph of the system is made up of 2 straight line graphs.
The graph of
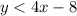 is a dashed straight line with shading under the line.
is a dashed straight line with shading under the line.
The graph of
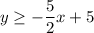 is a solid straight line with shading above the line.
is a solid straight line with shading above the line.
The solution area is the area where the shading of the two lines overlaps.
Part B
To determine if the point (5, -8) is included in the solution area, input the point into both inequalities:
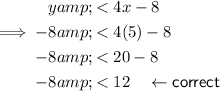
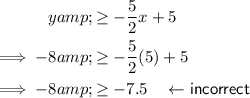
Therefore, the point (5, -8) is not included in the solution area as it is only true for one inequality.