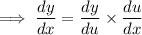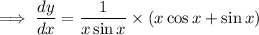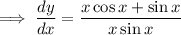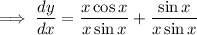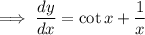Question

Please answer this question


​

Answer 1

`\displaystyle \large{(d)/(dx)[\log(x\sin x)] = (1)/(x\ln 10) + (\cot x)/(\ln 10)}`

Explanation:

Given:

`\displaystyle \large{y=\log (x\sin x)}`

Recall the (common) logarithmic differentiation & chain rules:

`\displaystyle \large{(d)/(dx)[\log (u)] = (u')/(u\ln 10)}`

Let
`\displaystyle \large{u=x\sin x}` then:

`\displaystyle \large{(d)/(dx)[\log(x\sin x)] = ((x\sin x)')/(x\sin x \ln 10)}`

Recall product rules:

`\displaystyle \large{[f(x)g(x)]' = f'(x)g(x)+f(x)g'(x)}`

Hence:

`\displaystyle \large{(d)/(dx)[\log(x\sin x)] = ((x)'\sin x + x(\sin x)')/(x\sin x \ln 10)}\\\\\displaystyle \large{(d)/(dx)[\log(x\sin x)] = (\sin x + x\cos x)/(x\sin x \ln 10)}\\\\\displaystyle \large{(d)/(dx)[\log(x\sin x)] = (\sin x)/(x \sin x \ln 10) + (x \cos x)/(x\sin x \ln 10)}\\\\\displaystyle \large{(d)/(dx)[\log(x\sin x)] = (1)/(x\ln 10) + (\cot x)/(\ln 10)}`

From above, recall that:

`\displaystyle \large{(d)/(dx)(\sin x) = \cos x}\\\\\displaystyle \large{(A\pm B)/(C) = (A)/(C) \pm (B)/(C)}\\\\\displaystyle \large{(\cos x)/(\sin x) =\cot x}`

Hence, the answer is:

`\displaystyle \large{(d)/(dx)[\log(x\sin x)] = (1)/(x\ln 10) + (\cot x)/(\ln 10)}`

Answer 2

`(dy)/(dx)=\cot x+(1)/(x)`

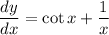
Explanation:

`\boxed{\begin{minipage}{6 cm}\underline{Product\:Rule}\\\\$(d)/(dx)[f(x)g(x)]=f(x)g'(x)+f'(x)g(x)$\\\\\\\underline{Chain\:Rule}\\\\$(dy)/(dx)=(dy)/(du) * (du)/(dx)$\\\end{minipage}}`

`\textsf{Given}:y=\log (x \sin x)`

`\textsf{Let }u=x \sin x \implies y=\log(u)`

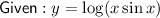
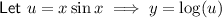
Using the product rule, differentiate y with respect to
`u`:

`\textsf{If }y=\log(u) \implies (dy)/(du)=(1)/(u)\implies (dy)/(du)=(1)/(x \sin x)`

----------------------------------------------------------------------------------------

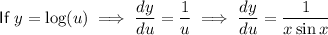
Differentiate
`u=x \sin x` with respect to x using the product rule:

`\implies \textsf{Let }f(x)=x \implies f'(x)=1`

`\implies \textsf{Let }g(x)=\sin x \implies g'(x)=\cos x`

`\implies (du)/(dx)=x \cos x + \sin x`

----------------------------------------------------------------------------------------

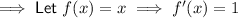
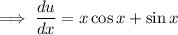
Use the chain rule to differentiate y with respect to x:

`\implies (dy)/(dx)=(dy)/(du) * (du)/(dx)`

`\implies (dy)/(dx)=(1)/(x \sin x) * (x \cos x + \sin x)`

`\implies (dy)/(dx)=(x \cos x + \sin x)/(x \sin x)`

`\implies (dy)/(dx)=(x \cos x)/(x \sin x)+(\sin x)/(x \sin x)`

`\implies (dy)/(dx)=\cot x+(1)/(x)`