Answer:
The standard deviation of the number of dogs who weigh 65 lbs or more is 1.83.
Explanation:
Let X represent the number of adult Australian sheep dogs weighing 65 pounds or more.
It is provided that the probability of occurrence of X is, p = 0.40.
A sample of n = 14 adult dogs is studied.
Each dog's weight is independent of the others.
The random variable X follows a Binomial distribution.
The standard deviation of a Binomial distribution is:
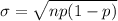
Compute the standard deviation of the number of dogs who weigh 65 lbs or more as follows:
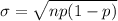
Thus, the standard deviation of the number of dogs who weigh 65 lbs or more is 1.83.