Answer:
All apply values are:
0 ⇒ A
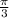 ⇒ B
⇒ B
 ⇒ E
⇒ E
Explanation:
∵ 0 ≤ x ≤ 2π is the domain for angle x
∴ 0 ≤
 ≤ π is the domain of angle
≤ π is the domain of angle
∵ sin(
 ) + cos(x) - 1 = 0
) + cos(x) - 1 = 0
→ To solve the equation we should use the rule of cosine double angle
∵ cos(x) = 1 - 2 sin²(
 )
)
→ Substitute it in the equation above
∴ sin(
 ) + (1 - 2 sin²(
) + (1 - 2 sin²(
 ) = 0
) = 0
∴ sin(
 ) + 1 - 2 sin²(
) + 1 - 2 sin²(
 ) = 0
) = 0
→ Add the like terms
∴ sin(
 ) + (1 - 1) - 2 sin²(
) + (1 - 1) - 2 sin²(
 ) = 0
) = 0
∴ sin(
 ) - 2 sin²(
) - 2 sin²(
 ) = 0
) = 0
→ Take sin(
 ) as a common factor
) as a common factor
∴ sin(
 ) [1 - 2sin(
) [1 - 2sin(
 )] = 0
)] = 0
→ Equate each factor by 0
∵ sin(
 ) = 0
) = 0
→ The value of sine equal zero on the x-axis
∴
 = 0, π, 2π
= 0, π, 2π
∵ The domain of
 is 0 ≤ (
is 0 ≤ (
 ) ≤ π
) ≤ π
∴
 = 0 and π ⇒ 2π refused because ∉ the domain
= 0 and π ⇒ 2π refused because ∉ the domain
→ Multiply both sides by 2 to find x
∴ x = 0 and 2π
∵ 1 - 2sin(
 ) = 0
) = 0
→ Subtract 1 from both sides
∴ - 2sin(
 ) = -1
) = -1
→ Divide both sides by -2
∴ sin(
 ) =
) =

→ The sine is positive in the 1st and 2nd quadrants
∴ (
 ) lies on the 1st OR 2nd quadrants
) lies on the 1st OR 2nd quadrants
∵ (
 ) =
) =

∴ (
 ) =
) =
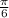 ⇒ 1st quadrant
⇒ 1st quadrant
→ Multiply both sides by 2
∴ x =
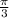
∵ (
 ) = π -
) = π -
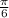 =
=
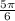 ⇒ 2nd quadrant
⇒ 2nd quadrant
→ Multiply both sides by 2
∴ x =

∴ The values of x are 0,
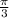 ,
,
 , 2π
, 2π
All apply values are:
0 ⇒ A
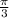 ⇒ B
⇒ B
 ⇒ E
⇒ E