Question

If 2000 ft³ of air is crossing an evaporator coil and is cooled from 80°F to 60°F, what would be the volume of air in ft³ exiting the evaporator coil?

Answer 1

Given :

If 2000 ft³ of air is crossing an evaporator coil and is cooled from 80°F to 60°F.

To Find :

The volume of air in ft³ exiting the evaporator coil.

Solution :

We know, relation between initial and final temperature and volume is given by :

`(V_1)/(V_2)=(T_1)/(T_2)`

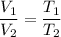
Here, temperature is in Kelvin .

We know, Kelvin temperature is given by :

`K=(F-32)* (5)/(9) + 273`

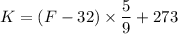
Putting F = 60° F,

`K=(60-32)* (5)/(9) + 273\\\\K = 288.56\ K`

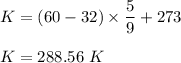
Putting F = 80° F,

`K=(80-32)* (5)/(9) + 273\\\\K = 299.67\ K`

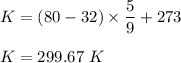
Putting all values, we get :

`(V_1)/(2000)=(60)/(80)\\\\V_1=(60* 2000)/(80)\ ft^3\\\\V_1=1500\ ft^3`

Hence, this is the required solution.