Answer:
rate = 7.580 ×
 /min
/min
Step-by-step explanation:
given data
value of n = 2.4
fraction recrystallized = 0.30
time = 100 min
solution
we will get here rate of recrystallization at this temperaturewe use here avrami equation that is
y = 1 - exp (-k
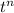 ) ................1
) ................1
we get hete first k
k =

put heer value n is 2.4 and y = 0.30 and t is 100
k =
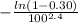
k = 5.65 ×

now we get here

value of t at y 0.5
 =
=
![[(-ln(1-y))/(k)]^(1/n)](https://img.qammunity.org/2021/formulas/engineering/college/t77tn6w82o88xbdkd42vhgqebgz60psqkz.png)
 =
=
![[\frac{-ln(1-0.5)}{5.65* 106{-6}}]^(1/2.4)](https://img.qammunity.org/2021/formulas/engineering/college/9lipzsee808cpejpewgnuf0yx9szedb1at.png)
 = 131.923 min
= 131.923 min
and
rate = 1 ÷

rate = 1 ÷ 131.923
rate = 7.580 ×
 /min
/min