Answer:
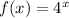 grows at a faster rate than the given quadratic function.
grows at a faster rate than the given quadratic function.
Explanation:
Given table:

First Differences in y-values:
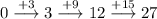
Second Differences in y-values:
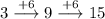
As the second differences are the same, the function is quadratic.
The coefficient of
 is always half of the second difference.
is always half of the second difference.
Therefore, the quadratic function is:

The average rate of change of function f(x) over the interval a ≤ x ≤ b is given by:

Therefore, the average rate of change for
 over the interval
over the interval
0 ≤ x ≤ 3 is:
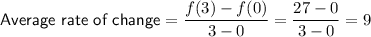
An exponential function that grows at a faster rate than
 over the interval 0 ≤ x ≤ 3 is
over the interval 0 ≤ x ≤ 3 is
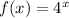
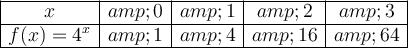

As 21 > 9,
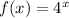 grows at a faster rate than
grows at a faster rate than
 over the interval 0 ≤ x ≤ 3.
over the interval 0 ≤ x ≤ 3.