Question

Find the values of x for which the function is continuous.

f(x) =

−3x + 7 if x < 0
x^2 + 7 if x ≥ 0

a.x ≠ 0

b.x ≥ 0


c.x ≥ root square 7
d.all real numbers

e.x ≠ 7

Answer 1

It looks like the given function is

`f(x) = \begin{cases}-3x + 7 & \text{if }x < 0 \\ x^2+7 & \text{if }x \ge0\end{cases}`

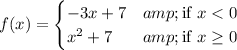
The two pieces of f(x) are continuous since they are polynomials, so we only need to worry about the point at which they meet, x = 0. f(x) is continuous there if

`\displaystyle \lim_(x\to0^-) f(x) = \lim_(x\to0^+) f(x) = f(0) = 7`

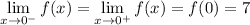
To the left of x = 0, we have x < 0, so f(x) = -3x + 7 :

`\displaystyle \lim_(x\to0^-) f(x) = \lim_(x\to0) (-3x + 7) = 7`

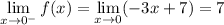
To the right of x = 0, we have x > 0 and f(x) = x² + 7 :

`\displaystyle \lim_(x\to0^+) f(x) = \lim_(x\to0) (x^2 + 7) = 7`

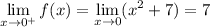
So f(x) is continuous at x = 0, and hence continuous for all real numbers.