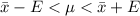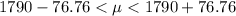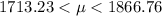Question

The cost of spare parts per machine are normally distributed with a population standard deviation of 158 dollars and an unknown population mean. If a random sample of 23 machines is taken and results in a sample mean of 1790 dollars, find the error bound (EBM) of the confidence interval with a 98% confidence level

Answer 1

The error bound (EBM) for the confidence interval with a 98% confidence level is approximately $59.88.

The error bound (EBM) for a confidence interval in a normal distribution is given by the formula:

EBM =
`Z * (\sigma)/(√(n))`

For a 98% confidence interval in a normal distribution, the Z-score is approximately 2.33.

Now, plug these values into the formula:

EBM =
`2.33 * (158)/(√(23))`

Calculating this, you get the error bound (EBM):

EBM
`\approx 2.33 * (158)/(√(23)) \approx 59.88 \]`

Therefore, the error bound (EBM) for the confidence interval with a 98% confidence level is approximately $59.88.

Answer 2

The 98% confidence interval is
`1713.23 <   \mu  <  1866.76`

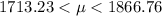
Explanation:

From the question we are told that

The population standard deviation is
`\sigma = 158`

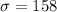
The sample size is n = 23

The sample mean is
`\= x = 1790 \ dollars`

From the question we are told the confidence level is 98% , hence the level of significance is

`\alpha = (100 - 98 ) \%`

=>
`\alpha = 0.02`

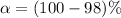
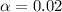
Generally from the normal distribution table the critical value of
`(\alpha )/(2)` is

`Z_{(\alpha )/(2) } =  2.33`

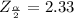
Generally the margin of error is mathematically represented as

`E = Z_{(\alpha )/(2) } *  (\sigma )/(√(n) )`

`E = 2.33 *  (158 )/(√(23) )`

`E =76.76`

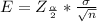
Generally 98% confidence interval is mathematically represented as

`\= x  -E <   \mu  <  \= x  +E`

`1790  -76.76 <  \mu  <  1790  +76.76`

`1713.23 <   \mu  <  1866.76`