Question

If the population variance of the lifetime of a light bulb is 10000 hours, how large of a sample must be taken in order to be 99% confident that the margin of error will not exceed 35 hours

Answer 1

The sample must be greater than 55

Explanation:

Confidence = 99% = 0.99

α = 1 - C = 1 - 0.99 = 0.01

α/2 = 0.01/2 = 0.005

The z score of α/2 (0.005) corresponds to the z score of 0.495 (0.5 - 0.005) which is equal to 2.576

`z_(\alpha)/(2) =2.576`

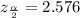
The margin of error (E) is given by the formula:

`E=z_(\alpha)/(2)*\sqrt{(\sigma^2)/(n) }\\ \\n=sample\ size,\sigma=standard\ deviation,\sigma^2=variance\\\\Given\ that:\\\\\sigma^2=10000,E=35,z_(\alpha)/(2)=2.576\\\\35= 2.576*\sqrt{(10000)/(n) }\\\\35= 2.576*(100)/(√(n) ) \\\\35= (257.6)/(√(n) )\\\\√(n) =(257.6)/(35 )\\\\√(n) =7.36\\\\n=7.36^2\\\\n=54.17`

n > 55

The sample must be greater than 55 so that the margin of error will not exceed 35 hours