Answer:
(a) 305.83 m/s
(b) +89° to the horizontal
(c) 305.83 m/s
(d)+89° to the horizontal
Step-by-step explanation:
The speed of the (air)planes = 165 m/s
The height from which the planes release the fuel tank = 3.38 km
The direction of one of the plane (plane A) at the time of release = 15° above the horizontal
The direction of the other plane (plane B) at the time of release = 15° below the horizontal
(a) The velocity of the plane is given as follows;
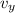 = v₀·sin(θ₀) - g·t
= v₀·sin(θ₀) - g·t
At maximum height,
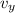 = 0
= 0
v₀·sin(θ₀) = g·t
t = 165×sin(15)/9.81 ≈ 4.35 s
The maximum height = 165×sin(15)×4.35 - 1/2×9.81×4.35 ² ≈ 92.95 m
The magnitude of the vertical velocity when the tank touches the ground is given as follows;
v² = u² - 2·g·s
u = 0
s = 3.38 + 0.09295 ≈ 3.47 km
v² = 2 × 9.81 × 3473
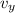 ≈ 261 m/s
≈ 261 m/s
The horizontal velocity = 165 × cos 15 ≈ 159.4 m/s
The magnitude = √(261² + 159.4²) ≈ 305.83 m/s
(b) The direction = tan^(-1)(261/159.4) ≈ 89° to the horizontal
(c) The time it takes the tank to reach the ground is given as follows;
v² = (165×sin(15))² + 2 × 9.81 × 3380 = 68139.33 m²/s²
v = 261.035 m/s
The horizontal velocity is the same as above = 159.4 m/s
The magnitude and direction will therefore be the same as for plane A which are
The magnitude = √(261² + 159.4²) ≈ 305.83 m/s
(d)The direction = tan^(-1)(261/159.4) ≈ 89° to the horizontal