Write both sides as powers of 16:
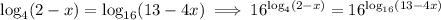
Since 16 = 4², we can rearrange the left side to get

Then, recalling that
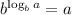 , we have
, we have

Now solve for x :
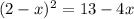
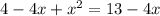
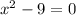
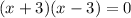
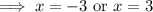
Notice that if x = 3, then log₄(2 - x) = log₄(-1) is undefined, so we throw out this solution. We don't run into this problem with x = -3, so that's the only (real) solution.