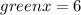Question

Use the angle relationship in the figure below to solve for x. Assume that line A and line Bare parallel, line C is a transversal and the given angles are given in

degrees
A
4x+29
12x+55
B

Answer 1

∠4x + 29° and angle ∠12x + 55° are interior angles on the same side of transversal . Which means their sum will be equal to 180° .

We can write this in an equation and solve it as :-

`\mapsto4x + 29 + 12x + 55 = 180`

`\mapsto \:16x + 29 + 55 = 180`

`\mapsto16x + 84 = 180`

`\mapsto16x = 180 - 84`

`\mapsto16x = 96`

`\mapsto \: x = (96)/(16)`

`\mapsto\color{darkorange} \: x = 6`

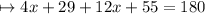
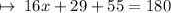
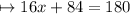
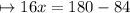
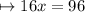
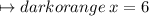
Let us check whether or not we have found out the correct value of x , by placing 6 in the place of x .

So :-

∠4x + 29° :-

`= 4 * 6 + 29`

`= 24 + 29`

`\color{red}= 53°`

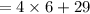
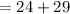
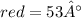
∠12x + 55° :-

`= 12 * 6 + 55`

`= 72 + 55`

`\color{teal} = 127°`

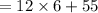
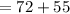
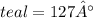
As , 53° + 127° = 180° , we can conclude that we have found out the correct value of x .

Therefore , the value of :-

`\color{green}x = 6`

Answer 2

x = 6

Explanation:

(4x + 29) + (12x + 55) = 180

16x +84 = 180

16x = 96

x = 6