Answer:
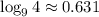
Explanation:
The change of base formula we can use is

Not all calculators will allow the user to directly enter the logarithms with a given base, so the change of base can be used for all calculators, which use the common log.
Common logs have a base of 10.
Let's convert your expression:
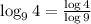
Using our calculator, we will compute log 4 divided by log 9:
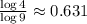 .
.