Question

A golf ball is hit horizontally off the edge of a 20 m high cliff and lands a distance of 18 m from the edge of the cliff. What was the initial vertical velocity of the ball?

0 m/s
16 m/s
-16 m/s
40 m/s

Answer 1

None of the options are correct.

Step-by-step explanation:

Let
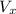`V_x` be the initial horizontal velocity of the golf ball t be the time of flight.

The time of flight, t, is the time taken by the ball to touch the ground. The ball falls towards earth due to gravitational force which acts vertically downward, so, the horizontal velocity of the ball remains unchanged.

As the horizontal distance covered by the ball is 18 m,

As distance = speed x time, so

`V_x t = 18\cdots(i)`

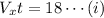
Now, by using the equation of motion in the gravitational field,

`s=ut+\frac 12 g t ^2`

Here, u is the initial velocity in the vertical direction, s is the displacement traveled in time t, g=9.81 is the acceleration due to gravity.

Here, we have u=0, s=20 m (height of the cliff),
`g=9.81 m/s^2`.

`\Rightarrow 20=0* t +\frac 12 (9.81)t^2`

`\Rightarrow t^2= \frac {20* 2}{9.81}`

`\Rightarrow t= \sqrt{\frac {40}{9.81}}`

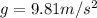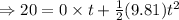
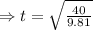
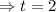`\Rightarrow t=2` seconds (approx)

From equation (i), e have

`V_x* 2=18`

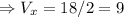`\Rightarrow V_x=18/2=9` m/s.

So, the initial velocity of the ball is 9m/s in the hprizontal diection.

Hence, none of the options are correct.