Question

Let f ( x ) = x 2 − 2 x f ( x ) = x 2 - 2 x . Round all answers to 2 decimal places. a . a . Find the slope of the secant line joining ( 2 , f ( 2 ) ( 2 , f ( 2 ) and ( 8 , f ( 8 ) ) ( 8 , f ( 8 ) ) .

Answer 1

The slope of the secant line joining (2, 0) and (8, 48) is 8.

Explanation:

Let
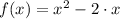`f(x) = x^(2)-2\cdot x`, from Analytical Geometry we remember that slope of a secant line is defined by:

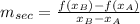`m_(sec) = (f(x_(B))-f(x_(A)))/(x_(B)-x_(A))` (Eq. 1)

Where:

`x_(A)`,
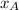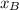`x_(B)` - Initial and final independent variables, dimensionless.

`f(x_(A))`,
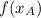`f(x_(B))` - Initial and final dependent variables, dimensionless.

Now we proceed to find the values of each dependent variable:

`x_(A) = 2`

`f(x_(A)) = 2^(2)-2\cdot (2)`

`f(x_(A)) = 0`

`x_(B) = 8`

`f(x_(B)) = 8^(2)-2\cdot (8)`

`f(x_(B)) = 48`

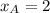
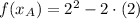
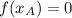
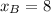
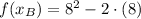
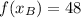
And slope of the secant slope is determined after replacing every variable:

`m_(sec) = (48-0)/(8-2)`

`m_(sec) = 8`

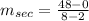
The slope of the secant line joining (2, 0) and (8, 48) is 8.