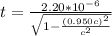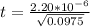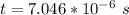Question

If such a particle is moving, with respect to the laboratory, with a speed of 0.950 c , what average lifetime is measured in the laboratory?

Answer 1

Complete Question

The positive
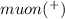`muon (^+)`, an unstable particle, lives on average
`2.20 * 10^(-6)\ s` (measured in its own frame of reference) before decaying.

If such a particle is moving, with respect to the laboratory, with a speed of 0.950 c , what average lifetime is measured in the laboratory?

Answer:

The value is
`\Delat  t  =  7.046 *10^(-6) \  s`

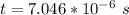
Step-by-step explanation:

From the question we are told that

The the average live time of
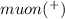`muon (^+)` is
`\Delta t_o  =  2.20 *10^(-6) \  s`

The speed of of
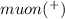`muon (^+)` in the laboratory is
`v  =  0.950 c`

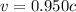
Generally the average life time of the positive
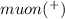`muon (^+)` measured in the laboratory is mathematically represented as

`\Delat  t  =  \frac{\Delta t_o }{ \sqrt{1 - (v^2)/(c^2) } }`

`\Delat  t  =  \frac{2.20 *10^(-6)}{ \sqrt{1 - ((0.950 c)^2)/(c^2) } }`

`\Delat  t  =  \frac{2.20 *10^(-6)}{ \sqrt{1 - (0.9025 c^2)/(c^2) } }`

`\Delat  t  =  (2.20 *10^(-6))/( √(1 - 0.9025  ) )`

`\Delat  t  =  (2.20 *10^(-6))/( √( 0.0975  ) )`

`\Delat  t  =  7.046 *10^(-6) \  s`