Question

If the profit function for a product is P(x) = 2400x + 30x2 − x3 − 26,000 dollars, selling how many items, x, will produce a maximum profit? x = items Find the maximum profit.

Answer 1

Selling 40 items will produce a maximum profit.

Explanation:

We need to use First and Second Derivative Tests on profit function to determine how many items will lead to maximum profit. Let
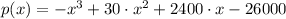`p(x) = -x^(3)+30\cdot x^(2)+2400\cdot x -26000`, where
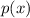`p(x)` is the profit for a product, measured in US dollars, and
`x` is the amount of items, dimensionless.

First we derive the profit function and equalize it to zero:

`p'(x) = -3\cdot x^(2)+60\cdot x+2400`

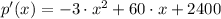
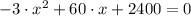`-3\cdot x^(2)+60\cdot x +2400 = 0` (Eq. 1)

Roots are found by Quadratic Formula:

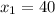`x_(1) = 40` and
`x_(2) = -20`

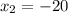
Only the first root may offer a realistic solution. The second derivative of the profit function is found and evaluated at first root. That is:

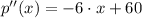`p''(x) = -6\cdot x +60` (Eq. 2)

`p''(40) = -6\cdot (40)+60`

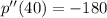`p''(40) = -180` (Absolute maximum)

Therefore, selling 40 items will produce a maximum profit.