Question

SP6 – A thin uniform rod is bent in the shape of a semicircle of radius R. Where is the center of mass?

Answer 1

Some coordinate (x, y)

Step-by-step explanation:

`x= (1)/(A) \int\limits^a_b {x(f(x)-g(x))} \, dx`

`y= (1)/(A) \int\limits^a_b {(1)/(2) (f(x)^2-g(x)^2)} \, dx`

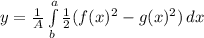
Those are the equations to find a centroid. Let's find the equation that defines the semicircle:

(usual circle, 1 is the radius)

`x^2 + y^2 = 1^2`

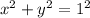
(this will give us our semicircle)

`y=√(1-x^2)`

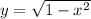
In the equation above, the difference between functions can be defined as a single function if we have no more than one.

Now all you need is to find the roots and compute the integrals.

Answer 2

Centroid of a semi circle is the center of the mass.

Step-by-step explanation:

Centroid is the point exactly at the center of the circle. If the rod is bent to form semi circle shape then its center of mass will be exactly at the centroid. The mass of the rod should be uniformly distributed. The rod shape should be symmetrical forming the semi circle.