Answer:
12.68 m (nearest hundredth)
Explanation:
Similar Triangle Theorem
If two triangles are similar, the ratio of their corresponding sides is equal.
Smaller triangle
- height = Ethan's height = 1.85 m
- base = 28.45 m - 24.3 m = 4.15 m
Larger triangle
- height = height of tree = h m
- base = 28.45 m
Ratio of height to base:



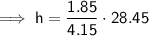
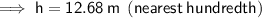
Therefore, the height of the tree to the nearest hundredth of a meter is 12.68 m.